All the Prism Geometry Information
An Elementary Math Student Requires

When your child actually starts referring to shapes as prisms, they most probably have already studied two prisms unknown to them.

The Cube which is a specific Cuboid, and a member of a sub group of polyhedra known as platonic solids, as all its faces are squares of equal size.

The cuboid is another name for a rectangular prism. 

In this section I cover everything your child needs to know about a prism.  I start with labeling the different parts of this shape, then give the formula needed to calculate both the surface area and volume of a prism. The next section gives diagrams and names of the different prisms your child should be exposed to.  

If you require more detailed information about any of these geometric shapes, simply click on the 'More Details' button.

Definition of a Prism

A prism is a three dimensional shape made up of two identical polygons, joined by straight lines.  A cross section of this shape will result in the exact shape of its two ends.

An important point to note here is that the ends are identical polygons.

A CONE is NOT a prism.

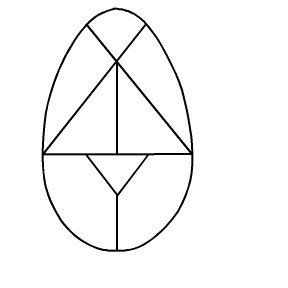
Parts of a Prism

Knowing how to label the different parts of a prism is an essential skill.

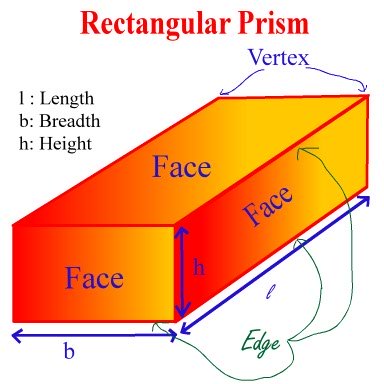Looking at this image, you can see there are only three measurements to any prism.

There are also terms that your child should be familiar with from their study of two dimensional shapes .

  • End: There are Two ends to a prism, both of which are identical in size and shape.
  • Vertex: The point where all the faces meet.
  • Length: The distance from the front face to the back face.
  • Breadth: Also known as width.  The distance from the left to right side of the front or back face.
  • Height: The perpendicular distance from the base to top.
  • Face: The surfaces of the prism.
  • Edge: The line where two or more faces meet.

Prism Formulae

Prism Surface Area

This is calculated by adding the area of each face to get the total surface area.

Because of the different number of faces, and depending on whether the prism is regular or not, dictates the formula. Get a more detailed surface area explanation here or explore each prism independently bellow.

Prism Volume

This is always calculated by multiplying the Area of the Base by the length of the prism. If you would like to read about this in more detail, read our volume of prism section.

Different Types of Prisms

There are as many different types of prisms, as there are polygons!  A prism is named after the shape of its front and back face. Bellow, I have listed the most commonly studied prisms at the elementary level.

Triangular Prism

S

S

Square Prism

S

S

Rectangular Prism

S

S

Pentagonal Prism

S

S

Hexagonal Prism

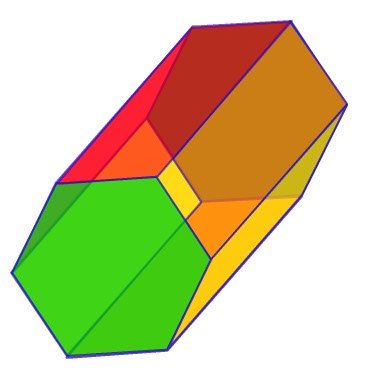S

S

Octagonal Prism

S

S

Regular and Irregular Prisms

Whether a Pyramid is Regular or Irregular is dictated by the type of Polygons it has as its front and back face.

Prisms created using a Regular Polygon as its front and back face is a Regular Prism.

An irregular Prism, is created by the use of irregular polygons as its front and back face.

Right and Oblique Prisms

This type of Prism is dictated by the vertices.

If the vertices of the front face are situated 90 degrees to their corresponding vertices of the back face, then it is a Right Prism.

If the vertex is situated ANYWHERE other than at 90 degrees, then it is an Oblique Prism.

Return from this Prism section and explore the other areas of 3d geometric shapes. 

Return to our K6 geometric shapes home page to explore more great basic geometry.

Enjoy this page? Please pay it forward. Here's how...

Would you prefer to share this page with others by linking to it?

  1. Click on the HTML link code below.
  2. Copy and paste it, adding a note of your own, into your blog, a Web page, forums, a blog comment, your Facebook account, or anywhere that someone would find this page valuable.

I am sure you will find all the information and worksheets you need here, however if there is anything you cannot find please don't hesitate to contact me or simply visit the MathMomentumCommunity and join the conversation!

I love to hear from my readers, and with a little feedback and a few suggestions I can make this a great resource for parents, teachers and tutors alike.

Be sure to explore everything on this site starting at the home page.