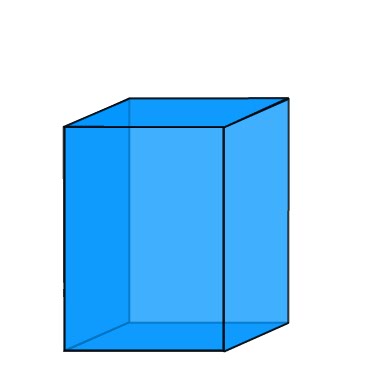
Triangular Pyramid
A one stop resource

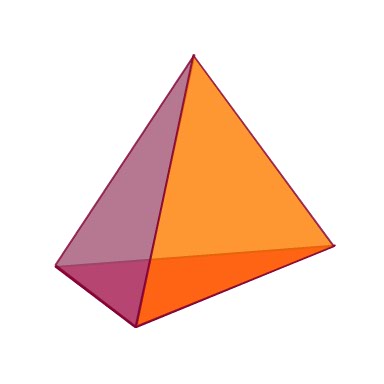
When people think of a triangular pyramid, most will automatically think of it as a type of Platonic Solid - the Tetrahedon. So I feel I must clarify this first.

Every tetrahedron is a triangular pyramid, but every triangular pyramid is NOT a tetrahedron. It is only a Platonic Solid if all it's faces are congruent, regular polygons, in this case

if every triangle is equilateral and equal in size.

Image

Base Shape

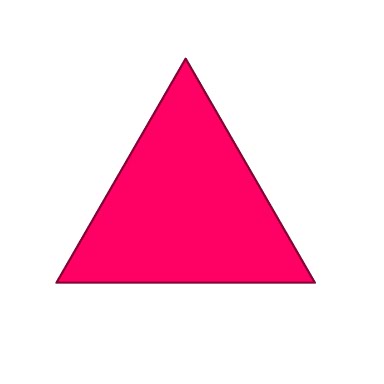Faces

Four

The three side faces are triangles and the base is also a triangle.

Vertices

4 Vertices

Edges

6 Edges

Surface Area

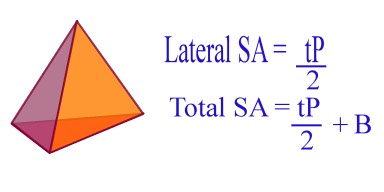Where: t = lateral height;

P = Perimeter of Base;

B = Area of Base

Volume

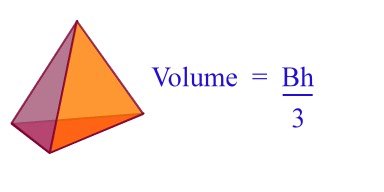I hope you find this resource helpful. If you have any comments or suggestions, on how I can make this geometry resource better for you

please don't hesitate to contact me.

Return from triangular pyramid to the main pyramid section.

or

Return to our k6 geometric shapes home page to explore more great basic geometry sections.

Enjoy this page? Please pay it forward. Here's how...

Would you prefer to share this page with others by linking to it?

  1. Click on the HTML link code below.
  2. Copy and paste it, adding a note of your own, into your blog, a Web page, forums, a blog comment, your Facebook account, or anywhere that someone would find this page valuable.

I am sure you will find all the information and worksheets you need here, however if there is anything you cannot find please don't hesitate to contact me or simply visit the MathMomentumCommunity and join the conversation!

I love to hear from my readers, and with a little feedback and a few suggestions I can make this a great resource for parents, teachers and tutors alike.

Be sure to explore everything on this site starting at the home page.